Resting potential

The resting membrane potential or just resting potential (abbreviated RMP or RP) is the membrane potential of excitable cells at rest, i.e. in non-excited nerve cells or muscle cells. A characteristic, temporary deviation from the resting potential is, for example, the action potential (AP) of these cells during excitation.

The resting potential corresponds in a good approximation to the diffusion potential of ions unequally distributed inside to outside the cells, primarily potassium (K+) next to sodium (Na+) and chloride (Cl-). It is more precisely determined by the sum of the respective equilibrium potentials taking into account the membrane conductivities for these ions (see also Goldman equation). The potential difference between the negatively charged cell interior and the extracellular environment across the membrane, called the resting membrane potential, is between -100 and -50 mV, depending on the cell type; in most nerve cells it is around -70 mV. This resting potential of an excitable cell is of fundamental cell physiological importance, among other things for the excitation conduction of the nerves, the control of muscle contraction, as well as the electrophoretic transport of substances across the membrane.

The membrane potential of many non-animal cells, such as those of plants, fungi or bacteria, is usually much more negative and is often around -200 mV due to the activity of a proton (H+) exporting ATPase (electrogenic pump). All living cells have a membrane potential, which is an electrical potential difference or voltage across the plasma membrane between the outside and inside of the cell. But only in excitable cells does its change from resting serve as a transmembrane signal.

Causes of the resting potential

There are four types of ions in the environment of the cell membrane, which are important for the formation of the resting potential: Na+, K+, Cl- and organic anions (A-), e.g. in the form of proteins.

Four factors are involved in the formation of the animal resting membrane potential:

1. Chemical gradient: particles move randomly and tend to be uniformly distributed (Brownian motion).
2. Electrical gradient: Voltage differences tend to equalize.
3. Selective permeability of the cell membrane: At rest (i.e. at the resting membrane potential), the cell membrane is permeable primarily to potassium ions (K+) and - depending on the cell type - to chloride ions (Cl-), less permeable to sodium ions (Na+) and practically impermeable to organic anions. Responsible for this are ion channels with specific conductivity for the different ions.
4. Sodium-potassium ion pump: the activity of sodium-potassium ATPase, an ion pump that pumps sodium ions out of the cell and potassium ions into the cell under ATP hydrolysis.

Since the electrical and the chemical gradient are two inseparable factors for the ion distribution, they are often summarized as electrochemical gradient.

The following factors continue to have an influence

• low, but nevertheless existing permeability of the membrane for sodium and calcium ions
• Permeability for chloride ions (Cl-).
• Self-protein synthesis of the cell (anionic proteins). Have effects on both the electrical and chemical gradient.

Diffusion potential

The phenomenon of diffusion potential is not limited to biology. The prerequisite is two compartments with different concentrations of a salt, for example a potassium salt, which are separated by a membrane permeable only to potassium (which can also be produced synthetically).

1. In the initial position, the vessel is electrically neutral because, although there are more potassium ions on one side, there are also more negatively charged counterions of the salt.
2. Only the potassium can pass through the membrane and will do so, in both directions. However, there are many more ions on one side than on the other, so that in total ions will pass from the high to the low concentration compartment. The driving force is the chemical potential due to a concentration gradient.
3. However, potassium ions have a charge. As soon as an ion crosses over, the second compartment is positively charged or the first negatively charged. There is an electric field, or what is equivalent, an electric potential difference or voltage across the membrane. This field exerts a force on the ions that drives them back against the chemical gradient.
4. An equilibrium is formed between the two forces, in which just as many particles diffuse in one direction as in the other per unit of time. This state is reached exactly when the energy expenditure for one path is equal to that of the other. If one equates the expressions for the electrical work and the chemical work along a concentration gradient, one obtains the Nernst equation.

The potential at this point is the equilibrium potential for the ion in question, in this case potassium.

Situation at the membrane

The biological membrane fulfils the requirements for a diffusion potential. The lipid bilayer is permeable for ions only to a very small extent. Transmembrane proteins are located in this layer, which are highly specific channels for the cations K+, Na+, Ca2+ or for anions. The opening of these channels can be controlled by various mechanisms, but these are not important for the resting potential.

Most channels are closed during the state of resting potential, only certain potassium channels are open (in humans, depending on the cell type, the group of voltage-independent potassium inward rectifier channels _Kir, the 2-P domain or background channels, and a voltage-dependent (KCNQ-type potassium) channel closing only at very negative voltages). Another transporter that is also open in the resting state is the sodium-potassium pump. Following the concentration gradient, small amounts of sodium ions constantly enter the cell from the outside and potassium ions from the inside into the extracellular space via leakage currents through the membrane. In contrast, the sodium-potassium pump (Na+-K+-ATPase) pumps 3 Na+ out and 2 K+ into the cell, consuming one ATP per transport cycle, thus establishing an electrochemical gradient (see below). The vast majority of the channels for sodium and calcium are closed.

The physiological concentrations of important ions in humans
Ion	Concentrationintracellular (mmol/l)	Concentrationextracellular (mmol/l)	Ratio	equilibrium potential according to Nernst
Na+	7–11	144	1:16	approx. +60 mV
K+	120–155	4–5	30:1	-91 mV
Ca2+	10−5–10−4	2		+125 mV to +310 mV
Cl-	4–7	120	1:20	-82 mV
HCO3-	8-10	26-28	1:3	-27 mV
H+	10-4 (pH 7.0)	4×10-5 (pH 7.4)	2,5:1	-24 mV
Anionic proteins	155	5

Ion imbalance

Large concentration gradients exist across the membrane of living cells for a number of ions. The concentration gradients, which are important for the resting membrane potential, are built up by the special nature of the membrane and the so-called sodium-potassium pump, an energy-dependent transport enzyme which, as a sodium-potassium ATPase, transports three Na+ ions out and two K+ ions in per split ATP molecule. In the balance, one positive charge is thus shifted across the membrane per cycle. The charge imbalance generated by the sodium-potassium ATPase is an important prerequisite for the resting membrane potential and essential for its maintenance.

Formation of the resting membrane potential

Now that a selectively permeable membrane and a concentration gradient are given, an equilibrium potential can develop.

Decisive for the resting membrane potential is the concentration gradient of the potassium ion. The resting membrane potential is determined by the equilibrium potential of the potassium ion.

This assertion is valid despite the fact that the resting membrane potential is never exactly at the value given by the Nernst equation for potassium ions. This is because the conductivity of the membrane for sodium and calcium ions is very low, but still not zero, and both ions are far from their equilibrium potential (see table), which means a high electrochemical driving force. Therefore, there are always sodium leakage currents (calcium to a lesser extent) into the cell interior, shifting the potential to positive and again driving potassium ions out of the cell. If the sodium-potassium ATPase did not constantly work against these leakage currents, the resting potential would soon be leveled.

The membrane is also permeable to chloride ions to a small extent. However, the equilibrium potential of the chloride ions is close to that for potassium ions. Nevertheless, the chloride ion also participates in the resting membrane potential.

Due to the involvement of other ions as well, the Nernst equation is not sufficient for an exact calculation. A better mathematical description is possible with the Goldman-Hodgkin-Katz equation, which includes not only potassium but also sodium and chloride ions in the calculation.

The above equations describe a steady state of the potential across the cell membrane, i.e. the resting membrane potential. However, if one considers the ability of some ion channels to change their conductance as a function of the applied voltage, the membrane conductance becomes a function of the voltage across the membrane and there is no longer a steady state. This is described in the Hodgkin-Huxley model, which describes the electrical states of one or more cells under different conditions.

The following can be summarized as causes for these gradients or as reasons for the prevention of a diffusion balance:

1. Different ion permeabilities across the membrane.
2. Immobility of intracellular proteins (Gibbs-Donnan effect; after Frederick George Donnan and Josiah Willard Gibbs).
3. Equilibrium potentials of ions (Nernst, Goldman).
4. Different conductivities for the respective ions.
5. The Na-K pump (electrogenic, concentration-shifting).

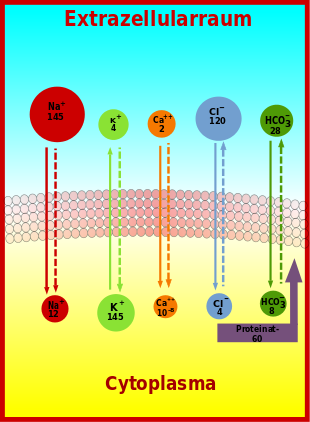
Plot of major ion gradients across the plasma membrane (solid arrows indicate the direction of the concentration gradient, dashed arrows indicate the direction of the potential gradient; Ca⁺⁺ concentration in the cytoplasm is given in moles per liter, all other concentrations in mmol/l).

Measurement of the resting membrane potential

The resting membrane potential can be determined experimentally with two microelectrodes. One of the two microelectrodes, the measuring electrode, is inserted into the cell, the second, the reference electrode, is held to the cell from the outside. On a voltmeter or cathode ray oscilloscope, a voltage (more precisely potential difference) in the order of -70 mV (many mammals) can be read between the electrodes: the resting potential. By definition, this voltage is the voltage difference across the membrane. The interior of the cell is negatively charged.

The measured values vary depending on the cell type and fluctuate between -50 and -100 mV. In human neurons the value is typically -70 mV, glial cells, cardiac and skeletal muscle cells show -90 mV, in smooth muscle the resting membrane potential is about -50 mV.