Density

This article represents the mass density. For other meanings, see Density (disambiguation). Words compounded with -density also denote other quantities related to volume, area, length, frequency interval, or other, see under the lemma of compound word.

The density ρ $\rho$ (Rho), also called mass density, is the quotient of the mass a body and its volume :

$\rho ={\frac {m}{V}}$ .

It is often expressed in grams per cubic centimetre or in kilograms per cubic metre. For liquid bodies, the unit kilogram per liter (= kilogram per cubic decimeter) is also common.

Density is determined by the material of the body and as an intensive quantity is independent of its shape and size.

In general, substances expand with increasing temperature, which causes their density to decrease. Substances with a density anomaly, such as water, are an exception.

Distinction from other terms

While density is the mass in relation to the volume, the weight indicates the weight force in relation to the volume, and is therefore dependent on the gravitational field.
The relative density is the ratio of the density to the density of a standard, i.e. a dimensionless quantity.

These differences are defined in DIN 1306 Density; terms, specifications. The density is a quotient quantity.

Determination of the density

buoyancy density

According to Archimedes' principle, a body completely immersed in a fluid (a liquid or a gas) experiences a buoyancy force equal to the weight force of the volume of the displaced substance. To determine the two unknowns density and volume, two measurements are required.

Immersing an arbitrary body of volume $V_{{\mathrm K}}$ completely into two fluids of known densities ρ $\rho _{1}$ and ρ $\rho _{2}$ , there are resultant forces $F_{1}$ and $F_{2}$ , which are measurable by means of a simple balance. The density ρ $\rho _{{\mathrm K}}$ of the body can be determined from this as follows:

Based on the formulas for the weight force $F_{{\mathrm G}}$ of the body and the buoyancy force $F_{{{\mathrm A}i}}$ the body in fluid

$F_{{\mathrm G}}=V_{{\mathrm K}}\cdot \rho _{{\mathrm K}}\cdot g$

$F_{{{\mathrm A}i}}=V_{{\mathrm K}}\cdot \rho _{i}\cdot g$

with gravitational acceleration a balance measures for the body immersed in fluid the force

$F_{i}=F_{{\mathrm G}}-F_{{{\mathrm A}i}}.$

From these two equations for the fluids ( i=1,2 ), one can eliminate the unknown volume $V_{{\mathrm K}}$ and obtain the solution:

$\rho _{{\mathrm K}}={\frac {F_{1}\cdot \rho _{2}-F_{2}\cdot \rho _{1}}{F_{1}-F_{2}}}$

If one density is much smaller than the other, ρ $\rho_1 \ll \rho_2$ (such as for air and water), the formula simplifies to:

$\rho _{{\mathrm K}}={\frac {F_{1}}{F_{1}-F_{2}}}\cdot \rho _{2}$

If one has only one liquid, say water with density ρ $\rho _{1}$ , the volume of the body can instead be determined by the volume of water displaced when fully immersed, for example by measuring the overflow from a full vessel with a graduated cylinder. From the above equation

$F_{1}=F_{{\mathrm G}}-F_{{{\mathrm A}1}}=V_{{\mathrm K}}\cdot g\cdot (\rho _{{\mathrm K}}-\rho _{1})$

is obtained by transforming:

$\rho _{{\mathrm K}}=\rho _{1}+{\frac {F_{1}}{V_{{\mathrm K}}\cdot g}}$

Archimedes already used this method to determine the density of the crown of a king, who doubted that it really consisted of pure gold (ρK = 19320 kg/m3).

The hydrometer (spindle) and Mohr's balance are based on this buoyancy weighing of liquids.

Other methods

Pycnometer, density determination of solids or liquids by measuring the displaced liquid volume
Isotope method, density determination by radiation absorption
Bending vibrator, density determination, especially of flowing liquid, by vibration measurement
Resistograph, density determination of wood via strength.
Suspension method, density determination by equilibrium determination with the aid of a heavy liquid

A simple estimate of the density can be obtained using the Girolami method.

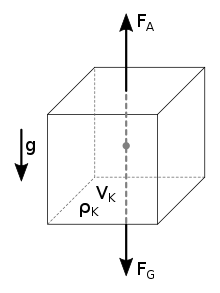
Forces acting on an immersed body

Questions and Answers

Q: What is density?

A: Density is a measurement that compares the amount of matter an object has to its volume.

Q: How is density measured?

A: Density is found by dividing the mass of an object by its volume.

Q: How is high density defined?

A: An object with much matter in a certain volume has high density.

Q: How is low density defined?

A: An object with little matter in the same amount of volume has a low density.

Q: What are the symbols used to represent density, mass, and volume?

A: The symbol used to represent density is ρ, the symbol used to represent mass is m, and the symbol used to represent volume is V.

Q: Can two objects with the same mass have different densities?

A: Yes, two objects with the same mass can have different densities if their volumes are different.

Q: What is the equation for finding density?

A: The equation for finding density is ρ = m/V, where ρ is the density, m is the mass, and V is the volume.